Tyco 579-769 specifications 104, Critical Polar Angle Calculations

Speaker Basics, Continued

Speaker Coverage,

(continued)

Determining Critical Polar Angle

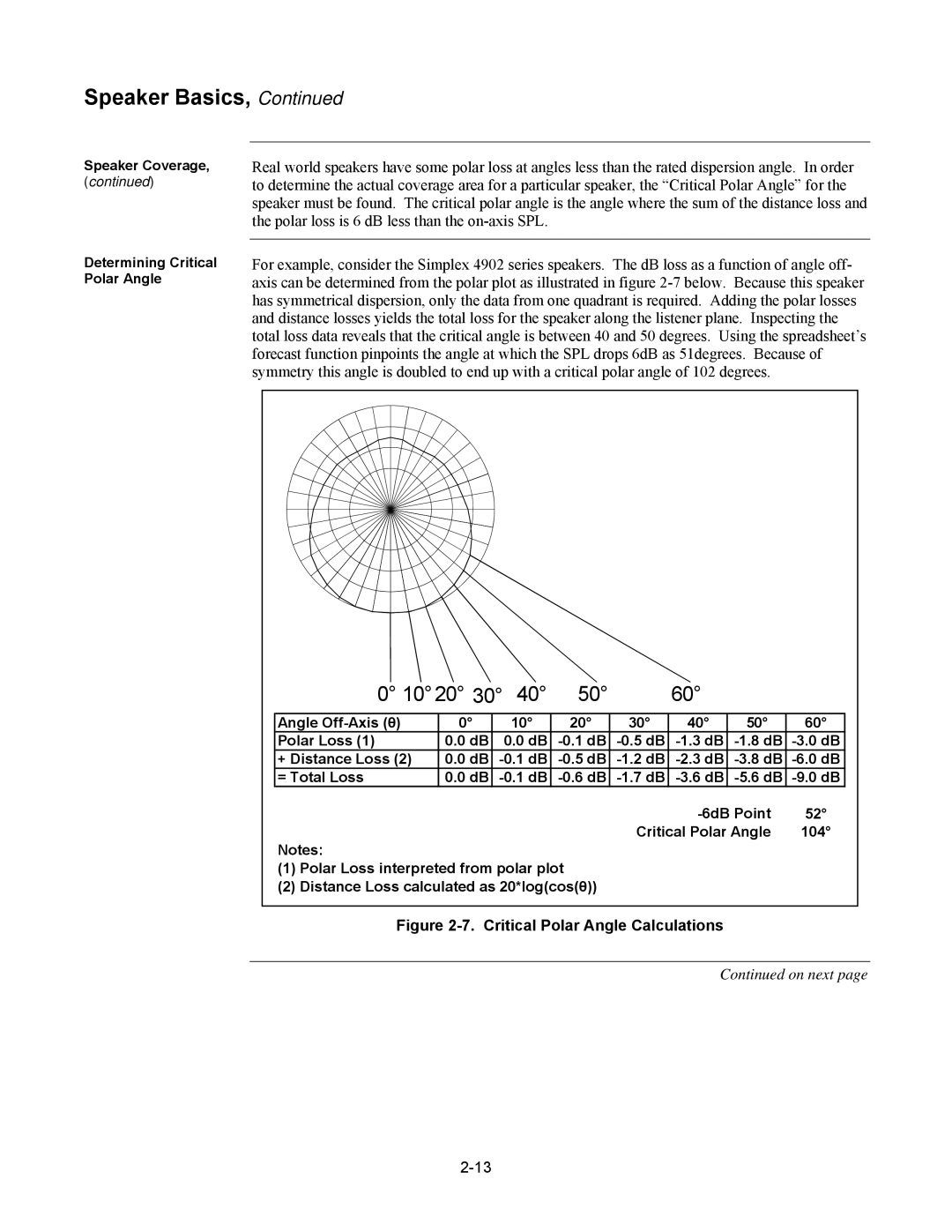
Real world speakers have some polar loss at angles less than the rated dispersion angle. In order to determine the actual coverage area for a particular speaker, the “Critical Polar Angle” for the speaker must be found. The critical polar angle is the angle where the sum of the distance loss and the polar loss is 6 dB less than the on-axis SPL.

For example, consider the Simplex 4902 series speakers. The dB loss as a function of angle off- axis can be determined from the polar plot as illustrated in figure 2-7 below. Because this speaker has symmetrical dispersion, only the data from one quadrant is required. Adding the polar losses and distance losses yields the total loss for the speaker along the listener plane. Inspecting the total loss data reveals that the critical angle is between 40 and 50 degrees. Using the spreadsheet’s forecast function pinpoints the angle at which the SPL drops 6dB as 51degrees. Because of symmetry this angle is doubled to end up with a critical polar angle of 102 degrees.

0° 10°20° 30°			40°	50°		60°
Angle Off-Axis (θ)	0°		10°	20°	30°	40°	50°	60°
Polar Loss (1)	0.0 dB		0.0 dB	-0.1 dB	-0.5 dB	-1.3 dB	-1.8 dB	-3.0 dB
+ Distance Loss (2)	0.0 dB	-0.1 dB		-0.5 dB	-1.2 dB	-2.3 dB	-3.8 dB	-6.0 dB
= Total Loss	0.0 dB	-0.1 dB		-0.6 dB	-1.7 dB	-3.6 dB	-5.6 dB	-9.0 dB

-6dB Point	52°
Critical Polar Angle	104°

Notes:

(1)Polar Loss interpreted from polar plot

(2)Distance Loss calculated as 20*log(cos(θ))

Figure 2-7. Critical Polar Angle Calculations

Continued on next page

2-13