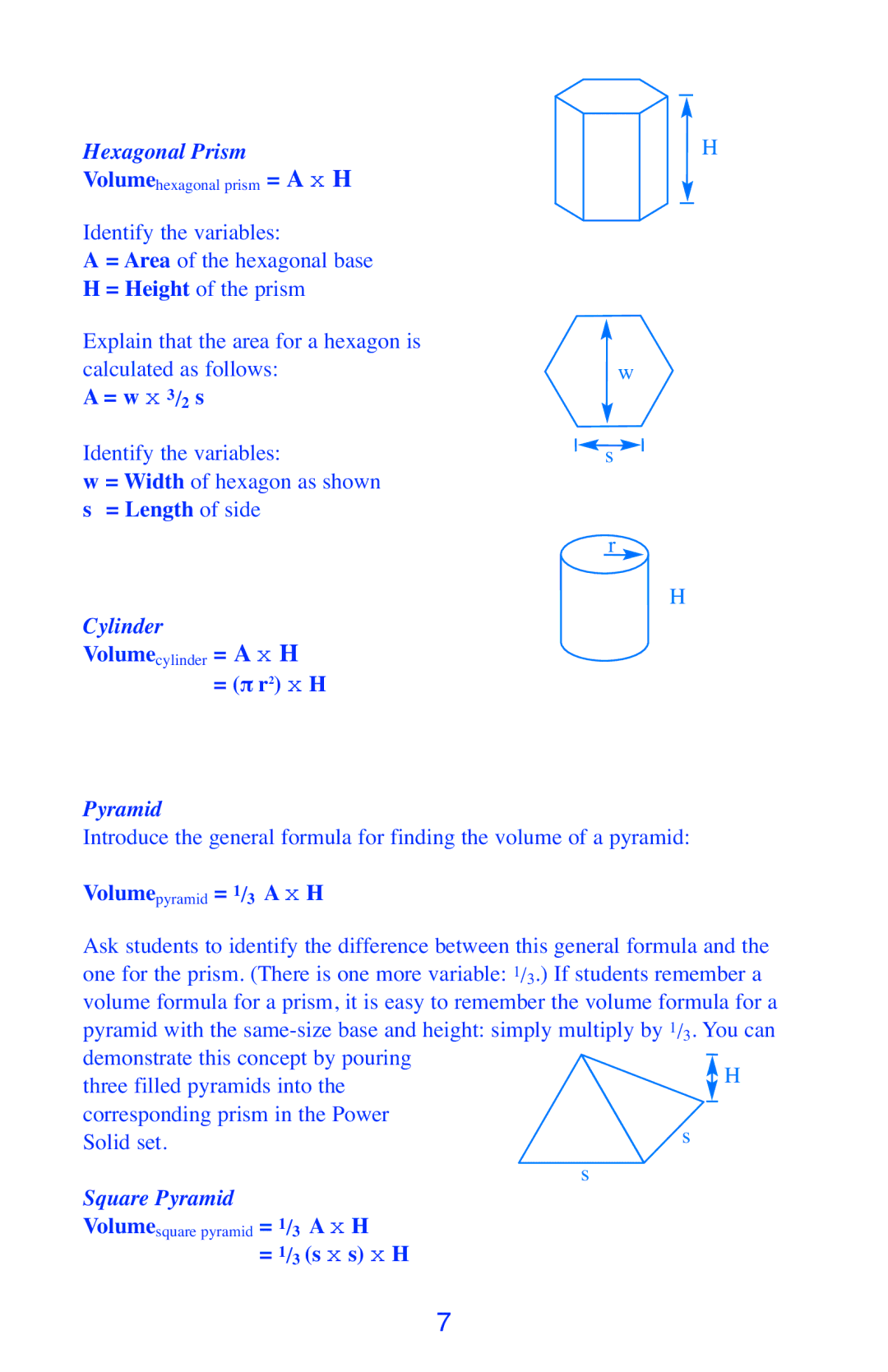
Hexagonal Prism A H
Volumehexagonal prism = x
Identify the variables:
A = Area of the hexagonal base H = Height of the prism
Explain that the area for a hexagon is calculated as follows:
A = w x 3/2 s
Identify the variables:
w = Width of hexagon as shown s = Length of side
Cylinder A H
Volumecylinder = x
= (π r2) x H
H
![]() w
w ![]() s
s ![]()
![]()
r
H
Pyramid
Introduce the general formula for finding the volume of a pyramid:
Volumepyramid = 1/3 A x H
Ask students to identify the difference between this general formula and the | |||||
one for the prism. (There is one more variable: 1/3.) If students remember a | |||||
volume formula for a prism, it is easy to remember the volume formula for a | |||||
pyramid with the | |||||
demonstrate this concept by pouring |
|
|
| H | |
three filled pyramids into the |
|
|
| ||
corresponding prism in the Power |
|
|
|
| |
| s | ||||
Solid set. |
| s | |||
Square Pyramid | A x H |
|
|
| |
|
|
|
| ||
Volumesquare pyramid = 1/3 |
|
|
|
| |
= 1/3 | (s x s) x H |
|
|
|
|
7