Agilent Technologies 8510-58 ∆∅radians = 2πf ∆length, Short circuit inductance L0 , L1, L2 and L3

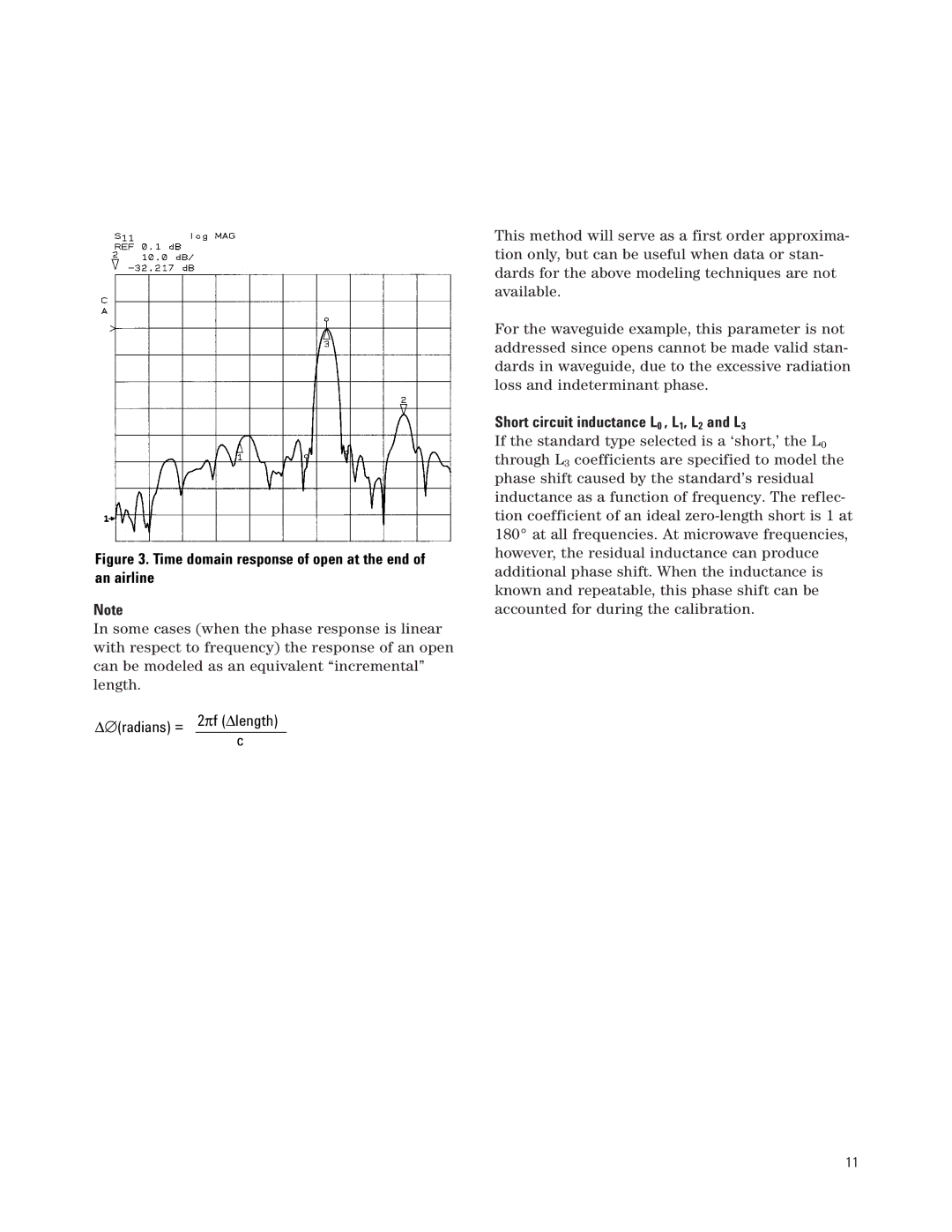
Figure 3. Time domain response of open at the end of an airline

Note

In some cases (when the phase response is linear with respect to frequency) the response of an open can be modeled as an equivalent “incremental” length.

∆∅(radians) = 2πf (∆length)

c

This method will serve as a first order approxima- tion only, but can be useful when data or stan- dards for the above modeling techniques are not available.

For the waveguide example, this parameter is not addressed since opens cannot be made valid stan- dards in waveguide, due to the excessive radiation loss and indeterminant phase.

Short circuit inductance L0 , L1, L2 and L3

If the standard type selected is a ‘short,’ the L0 through L3 coefficients are specified to model the phase shift caused by the standard’s residual inductance as a function of frequency. The reflec- tion coefficient of an ideal zero-length short is 1 at 180° at all frequencies. At microwave frequencies, however, the residual inductance can produce additional phase shift. When the inductance is known and repeatable, this phase shift can be accounted for during the calibration.

11