HP 50g manual Polar representation of a complex number

Polar representation of a complex number

The polar representation of the complex number 3.5-1.2i, entered above, is obtained by changing the coordinate system to cylindrical or polar (using function CYLIN). You can find this function in the catalog (‚N). You can also change the coordinate to polar using H. Changing to polar coordinate with standard notation and the angular measure in radians, produces the result in RPN mode:

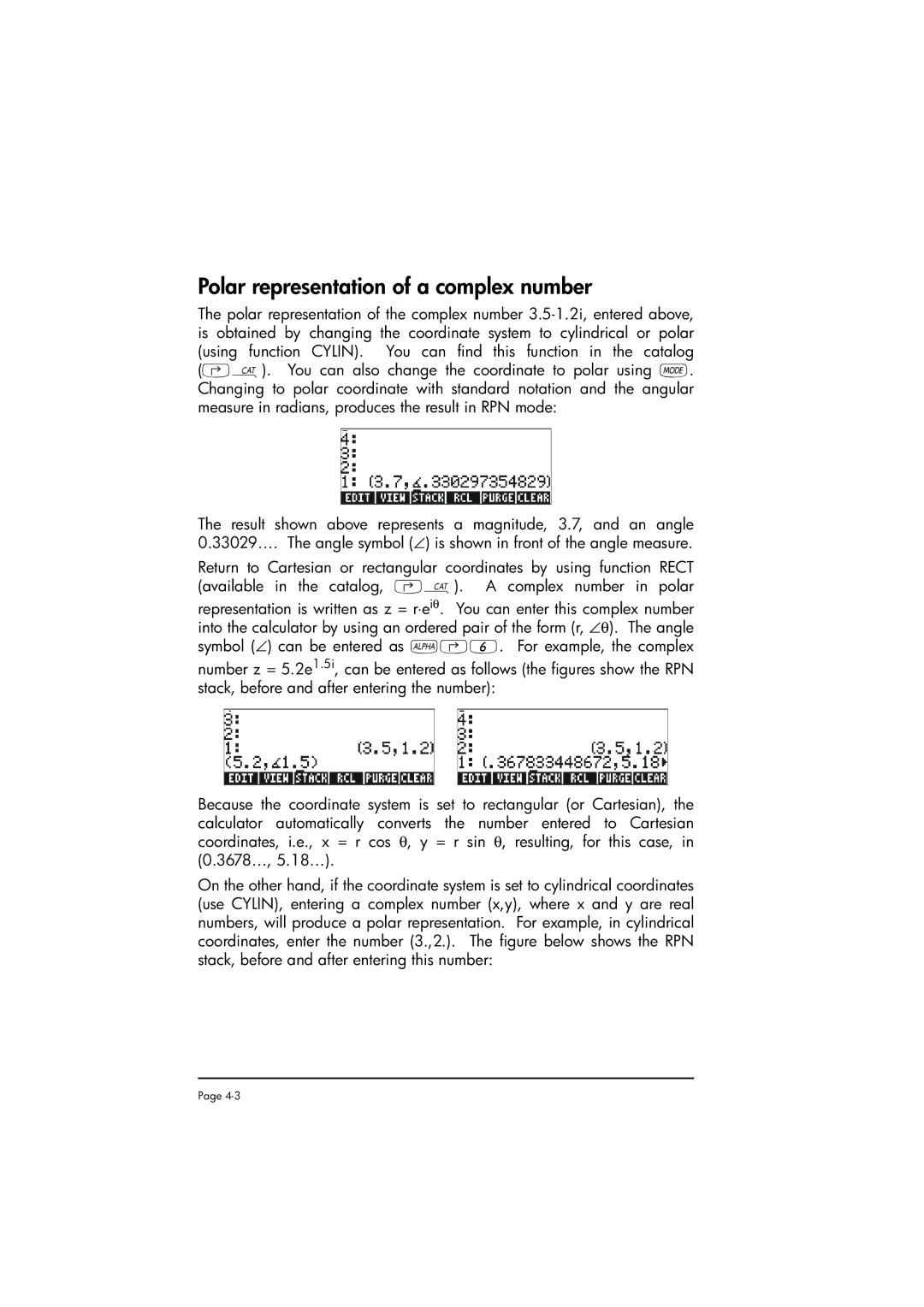
The result shown above represents a magnitude, 3.7, and an angle 0.33029…. The angle symbol (∠) is shown in front of the angle measure.

Return to Cartesian or rectangular coordinates by using function RECT (available in the catalog, ‚N). A complex number in polar

representation is written as z = r⋅eiθ. You can enter this complex number into the calculator by using an ordered pair of the form (r, ∠θ). The angle symbol (∠) can be entered as ~‚6. For example, the complex number z = 5.2e1.5i, can be entered as follows (the figures show the RPN stack, before and after entering the number):

Because the coordinate system is set to rectangular (or Cartesian), the calculator automatically converts the number entered to Cartesian coordinates, i.e., x = r cos θ, y = r sin θ, resulting, for this case, in (0.3678…, 5.18…).

On the other hand, if the coordinate system is set to cylindrical coordinates (use CYLIN), entering a complex number (x,y), where x and y are real numbers, will produce a polar representation. For example, in cylindrical coordinates, enter the number (3.,2.). The figure below shows the RPN stack, before and after entering this number:

Page 4-3