because it shows why a wave that travels twice as far as another wave of equal magnitude is not half as strong. Take the following example:
Two transmitters TXA and TXB both emit signals that are exactly the same at 1 Watt of power. The signal from TXA travels 10 units. Power at that point can be calculated by 1/102 x 1W or 0.01 x 1W. That means there is 0.01W of the TXA signal left after it has traveled 10 units. Now let’s say that the TXB signal travels twice the distance of TXA or 20 units. Power at that point can be calculated by 1/202 x 1W or 0.0025 x 1W. That means there is 0.0025W of the TXB signal left after it has traveled 20 units. As you can see, the signal that traveled twice as far was not ½ the power, but ¼ the power of the first signal.
Because of the inverse square law, the effective radiated power (ERP) of a given transmitter must increase by a factor of four times to achieve twice the operating range. This information is important in determining the necessary power for a wireless system for a given range. It is always important to use the minimum power necessary to accomplish the task at hand so that excess power does not affect other systems and cause undue harm.
The theoretical range of an RF system is important to know, but it is the functional range you must be more concerned with. The functional range of a system takes into account a certain cushion factor called fade margin that will ensure the signal coming from the transmitter to the receiver will not only be detectable, but will also be usable. This is less of a concern in communications systems as you can tolerate less fade margin than in an
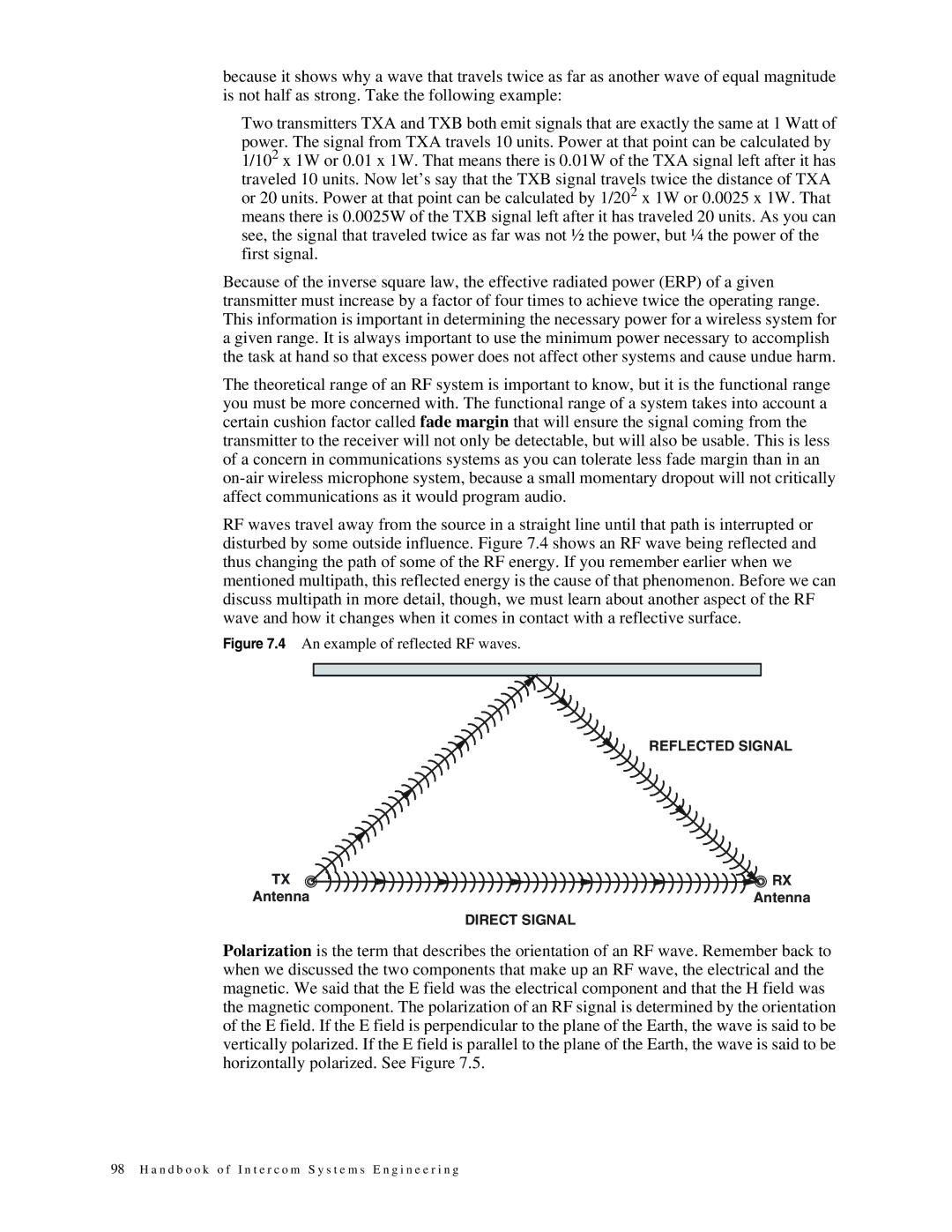
RF waves travel away from the source in a straight line until that path is interrupted or disturbed by some outside influence. Figure 7.4 shows an RF wave being reflected and thus changing the path of some of the RF energy. If you remember earlier when we mentioned multipath, this reflected energy is the cause of that phenomenon. Before we can discuss multipath in more detail, though, we must learn about another aspect of the RF wave and how it changes when it comes in contact with a reflective surface.
Figure 7.4 An example of reflected RF waves.
REFLECTED SIGNAL
TX | RX |
Antenna | Antenna |
DIRECT SIGNAL
Polarization is the term that describes the orientation of an RF wave. Remember back to when we discussed the two components that make up an RF wave, the electrical and the magnetic. We said that the E field was the electrical component and that the H field was the magnetic component. The polarization of an RF signal is determined by the orientation of the E field. If the E field is perpendicular to the plane of the Earth, the wave is said to be vertically polarized. If the E field is parallel to the plane of the Earth, the wave is said to be horizontally polarized. See Figure 7.5.
98 H a n d b o o k o f I n t e r c o m S y s t e m s E n g i n e e r i n g