Philips Semiconductors
Magnetoresistive sensors for
magnetic field measurement
General
APPENDIX 1: THE MAGNETORESISTIVE EFFECT
Magnetoresistive sensors make use of the fact that the electrical resistance ρ of certain ferromagnetic alloys is influenced by external fields. This
Resistance- field relation
The specific resistance ρ of anisotropic ferromagnetic
metals depends on the angle Θ between the internal |
|
magnetization M and the current I, according to: |
|
ρ(Θ) = ρ⊥ + (ρ⊥ − ρ) cos2 Θ | (1) |
where ρ⊥ and ρare the resistivities perpendicular and parallel to M. The quotient (ρ⊥ − ρ)/ρ⊥ = Δρ/ρ
is called the magnetoresistive effect and may amount to several percent.
Sensors are always made from ferromagnetic thin films as this has two major advantages over bulk material: the resistance is high and the anisotropy can be made uniaxial. The ferromagnetic layer behaves like a single domain and has one distinguished direction of magnetization in its plane called the easy axis (e.a.), which is the direction of magnetization without external field influence.
handbook, halfpage |
|
| L |
|
|
|
|
| ||
|
|
|
|
|
|
| ||||
|
|
|
|
|
|
|
|
| ||
y |
| M |
|
|
|
| ||||
| ϕ |
|
|
|
| |||||
|
|
|
|
|
| ϑ | Ι | W | ||
|
|
|
|
|
| |||||
|
|
|
|
|
|
|
| |||
|
|
|
|
|
|
|
| |||
|
|
|
|
|
|
|
|
| ||
|
|
|
|
|
|
|
|
|
|
|
|
| x |
|
|
| MBH616 | ||||
|
|
|
|
| ||||||
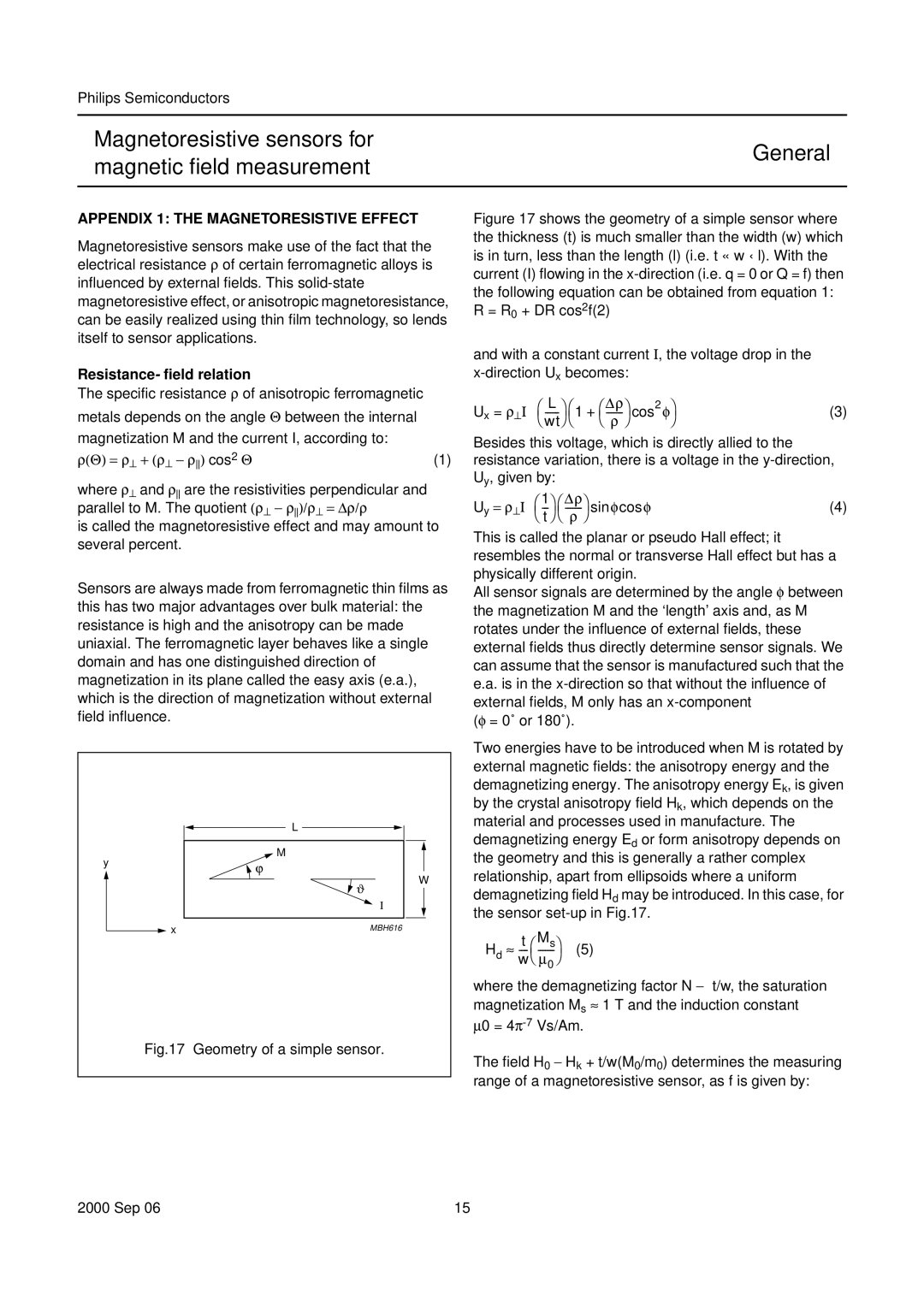
Fig.17 Geometry of a simple sensor.
Figure 17 shows the geometry of a simple sensor where the thickness (t) is much smaller than the width (w) which is in turn, less than the length (l) (i.e. t « w ‹ l). With the current (I) flowing in the x-direction (i.e. q = 0 or Q = f) then the following equation can be obtained from equation 1: R = R0 + DR cos2f(2)
and with a constant current Ι, the voltage drop in the x-direction Ux becomes:
Ux = ρ⊥Ι | ⎛ L | ⎞ ⎛ | 1 | + | ⎛ | Δρ⎞ | cos | 2 | ⎞ | (3) | |
| ⎝ | ⎠ |
| φ | |||||||
| ⎝ wt⎠ ⎝ |
|
| ρ |
|
| ⎠ |
| |||
Besides this voltage, which is directly allied to the resistance variation, there is a voltage in the
Uy = ρ⊥Ι | ⎛ 1⎞ ⎛ | Δρ⎞ | sinφcosφ | (4) | ||
⎠ ⎝ | ⎠ | |||||
| ⎝ t | ρ |
|
| ||
This is called the planar or pseudo Hall effect; it resembles the normal or transverse Hall effect but has a physically different origin.
All sensor signals are determined by the angle φ between the magnetization M and the ‘length’ axis and, as M rotates under the influence of external fields, these external fields thus directly determine sensor signals. We can assume that the sensor is manufactured such that the e.a. is in the
(φ = 0˚ or 180˚).
Two energies have to be introduced when M is rotated by external magnetic fields: the anisotropy energy and the demagnetizing energy. The anisotropy energy Ek, is given by the crystal anisotropy field Hk, which depends on the material and processes used in manufacture. The demagnetizing energy Ed or form anisotropy depends on the geometry and this is generally a rather complex relationship, apart from ellipsoids where a uniform demagnetizing field Hd may be introduced. In this case, for the sensor
H ≈ t ⎛ Ms⎞ (5)
d w μ0
where the demagnetizing factor N − t/w, the saturation magnetization Ms ≈ 1 T and the induction constant μ0 =
The field H0 − Hk + t/w(M0/m0) determines the measuring range of a magnetoresistive sensor, as f is given by:
2000 Sep 06 | 15 |