HP-15C Owner’s Handbook
HP Part Number 00015-90001 Edition 2.4, Sep
Legal Notice
Introduction
Contents
Contents
Display and Continuous Memory
Program Editing
Program Branching and Controls
Subroutines
Indirect Display Control
Calculating With Complex Numbers
Calculating With Matrices
Numerical Integration
Contents Appendix a Error Conditions
Appendix C Memory Allocation
Appendix D a Detailed Look at
Appendix E a Detailed Look at f
Contents Appendix F Batteries
Function Summary and Index
Programming Summary and Index
Subject Index
HP-15C Problem Solver
Quick Look at
Manual Solutions
To Compute Keystrokes Display
Programmed Solutions
Keystrokes Display
KeystrokesDisplay
001-42,21,11
002 003 004 005 006 007 008 009 8313
300.51
HP-15C a Problem Solver
Part l HP-15C Fundamentals
Power On and Off
Getting Started
Keyboard Operation
Section
Prefix Keys
Changing Signs
Keying in Exponents
I O m ´ P I l F T s ? t H b
Clear Keys
Display Clearing ` and −
Digit entry not terminated
Clears only the last digit
Calculations
One-Number Functions
Two-Number Functions
6532
17 +
26.0000 22.0000 5000
13.0000
78.0000
Numeric Functions
Number Alteration Functions
One-Number Functions
General Functions
Trigonometric Operations
Time and Angle Conversions
Pressing Calculates
Degrees/Radians Conversions
7069
Radians
40.5000
Logarithmic Functions
Hyperbolic Functions
Power Function
Two-Number Functions
Percentages
To Calculate Keystrokes Display
Polar and Rectangular Coordinate Conversions
Enters the base number the price
Calculates 3% of $15.76 the tax
Polar Conversion. Pressing
Keystrokes Display
Automatic Memory Stack Last X, and Data Storage
Automatic Memory Stack Stack Manipulation
Automatic Memory Stack Registers
Always displayed
Stack Manipulation Functions
Memory Stack, Last X, and Data Storage
Lost
Lost
Last X Register and K
287.0000
22.2481
12.9000
Calculator Functions and the Stack
13.9 +
20.6475
Order of Entry and the v Key
+15 X15
Nested Calculations
7 +
65.0000
69.0000
Arithmetic Calculations With Constants
5 ‛15 Keys
Keystrokes Display Growth factor
1000
000
Storage Register Operations
Storing and Recalling Numbers
322.5000
520.8750
Clearing Data Storage Registers
Storage and Recall Arithmetic
For storage arithmetic
For recall arithmetic
Problems
Overflow and Underflow
24 l-0
15.0000
Memory Stack, Last X, and Data Storage
Statistics Functions
Probability Calculations
60.0000
Random Number Generator
270,725.0000
5764
3422
Accumulating Statistics
Registers
Register Contents
20.00 40.00 60.00 80.00 Kg per hectare
Metric tons per Hectare, y
20 z 61v 40 z 7.21 60 z 7.78 80 z l
Σy2
Correcting Accumulated Statistics
20 w 20 z
Mean
Standard Deviation
40.00
Linear Regression
31.62
Standard deviation about the mean nitrogen
Application
Linear Estimation and Correlation Coefficient
Statistics Functions
Other Applications
70 ´j
Display Continuous Memory
Display Control
Fixed Decimal Display
Scientific Notation Display
Engineering Notation Display
234568
234567
Round-Off Error
Special Displays
Mantissa Display
Annunciators
Error Display
Digit Separators
12,345.67
12.345.6700
Low-Power Indication
Continuous Memory
Status
Resetting Continuous Memory
Page
Part ll HP-15C Programming
Programming Basics
Mechanics
Creating a Program
Loading a Program
Programming Basics
´b a
Intermediate Program Stops
Running a Program
002 003 004 005 006 007 008
How to Enter Data
300.51 300.51 ´A
Program Memory
Radius, r Height, h Base Area Volume Surface Area
Totals
002
004 005
007-44,40
010
Or G a
Further Information
Program Instructions
Instruction Coding
Memory Configuration
Keycode 25 second row, fifth key
Initial Memory Configuration
60 ´ m%
Program Boundaries
´ m %
19 ´ m%
19.0000
Unexpected Program Stops
Abbreviated Key Sequences
´bA ´b3 End of memory
User Mode
Polynomial Expressions and Horners Method
¤ @ y ∕
LOG %
Nonprogrammable Functions
001-42,21,12
002 003 004 005 006 007 008 009 0000
12,691.0000
Problems
Program Editing
Moving to a Line in Program Memory
Examples
Deleting Program Lines
Inserting Program Lines
Or use Â
Single-Step Operations
Line Position
Âhold
Release
Result
Insertions and Deletions
Initializing Calculator Status
Interest
+ i n
PV 1 + i n
100 270
´bA D ´4 O0 2* O1 2÷ * ´ ´ l0 l1 ´r * n
Program Branching Controls
Branching
Conditional Tests
Test
Flags
n will clear flag number n
Example Branching and Looping
010-45,20
013-43,30
014
016-44,40
Example Flags
Formula is
002-43
004-42,21,15
005-43, 4
006-42,21
Go to
250.0000
48.0000
10,698.3049
Looping
Conditional Branching
System Flags Flags 8
Program Branching and Controls
Subroutines
Go To Subroutine and Return
Subroutine Execution
´b.1
Subroutine Limits
000 001- ´b9
002- R
003- O0
004
´ b.4
´b.5
Subroutine Return
Nested Subroutines
Index Register Loop Control
V and % Keys
106
Indirect Program Control With the Index Register
Program Loop Control
Index Register Storage and Recall
Index Register and Loop Control
Index Register Arithmetic
Exchanging the X-Register
Indirect Branching With
Indirect Flag Control With
Indirect Display Format Control With
Loop Control With Counters I and e
Nnnnn x x x y y 5 0 0
Start count at zero Count by twos Count up to
Examples Register Operations
Iterations
Storing and Recalling Keystrokes Display
12.3456
Example Loop Control with e
Exchanging the X-Register
Storage Register Arithmetic
Loop control number in R2
−− 011- 42
012-42, 5
013- 22
Example Display Format Control
15 O
64.8420 0000 50.0000
Index Register Contents
Indirect Display Control
Index Register and Loop Control
118
Part lll HP-15C Advanced Functions
Complex Stack and Complex Mode
Calculating With Complex Numbers
Creating the Complex Stack
120
Deactivating Complex Mode
Complex Numbers and the Stack
Entering Complex Numbers
´ % hold 8.0000 release
Z 8 Y 7 X Keys
Stack Lift in Complex Mode
Manipulating the Real and Imaginary Stacks
Clearing a Complex Number
Or other operation
Continue with any operation
− 4 v Continue with any operation
Entering Complex Numbers with −. The clearing functions −
´ %hold release
0000 17.0000 144.0000
Entering a Real Number
Followed by another number
Entering a Pure Imaginary Number
´ Continue with any operation
Operations With Complex Numbers
Storing and Recalling Complex Numbers
´ O
L 2 ´
¤x N o ∕ @ a
+ * ÷ y
2000
7000
0428
0491
Polar and Rectangular Coordinate Conversions
Complex Results from Real Numbers
5708
´ % hold Release1.5708
Cos θ + i sin θ = re iθ Polar + ib = ∠ θ
8452
2981
+ 3.1434
352.0000
872.0000
2361
4721
For Further Information
Calculating With Matrices
138
Keystrokes Display Deactivates Complex Mode
= A-1B
Matrix Dimensions
Running
11.2887
2496
Dimensioning a Matrix
Number Rows Columns
Displaying Matrix Dimensions
Changing Matrix Dimensions
´mA
Keystrokes l B Display
Storing and Recalling Matrix Elements
Storing and Recalling All Elements in Order
⎡ a
Checking and Changing Matrix Elements Individually
Keystrokes Display
Matrix Operations
Storing a Number in All Elements of a Matrix
Matrix Descriptors
Result Matrix
Copying a Matrix
One-Matrix Operations
Calculating with Matrices
Scalar Operations
LB b
Elements of Result Matrix
LA a
Arithmetic Operations
Keystrokes Display Subtracts 1 from the elements
LB b 2 LA a 2
Matrix Multiplication
= AT B
Keystrokes Display l a a
Solving the Equation AX = B
24 OA
2400
86 OA
8600
274 OB 233 OB 331 OB 120.32 OB 112.96 OB 151.36 OB ´Á
Calculating the Residual
Week Cabbage kg 186 141 215 Broccoli kg 116
Using Matrices in LU Form
Calculations With Complex Matrices
Storing the Elements of a Complex Matrix
Then Z can be represented in the calculator by
Pressing Transforms Into
= ⎢
LA a
Complex Transformations Between ZP and Z
Inverting a Complex Matrix
Multiplying Complex Matrices
´ a
Keystrokes lA lB Display Displays descriptor of matrix a
´U lC LC lC lC lC lC lC lC ´U
Solving the Complex Equation AX = B
ZZ −1
AX = B
200.0000
170.0000
0372
1311
0437
1543
Calculating with Matrices
Using a Matrix Element With Register Operations
Using Matrix Descriptors in the Index Register
Miscellaneous Operations Involving Matrices
Stack Operation for Matrix Calculations
Conditional Tests on Matrix Descriptors
Calculating with Matrices
Using Matrix Operations in a Program
Summary of Matrix Functions
Keystrokes Results
´m a
Calculates residual in result matrix
For Further Information
Using
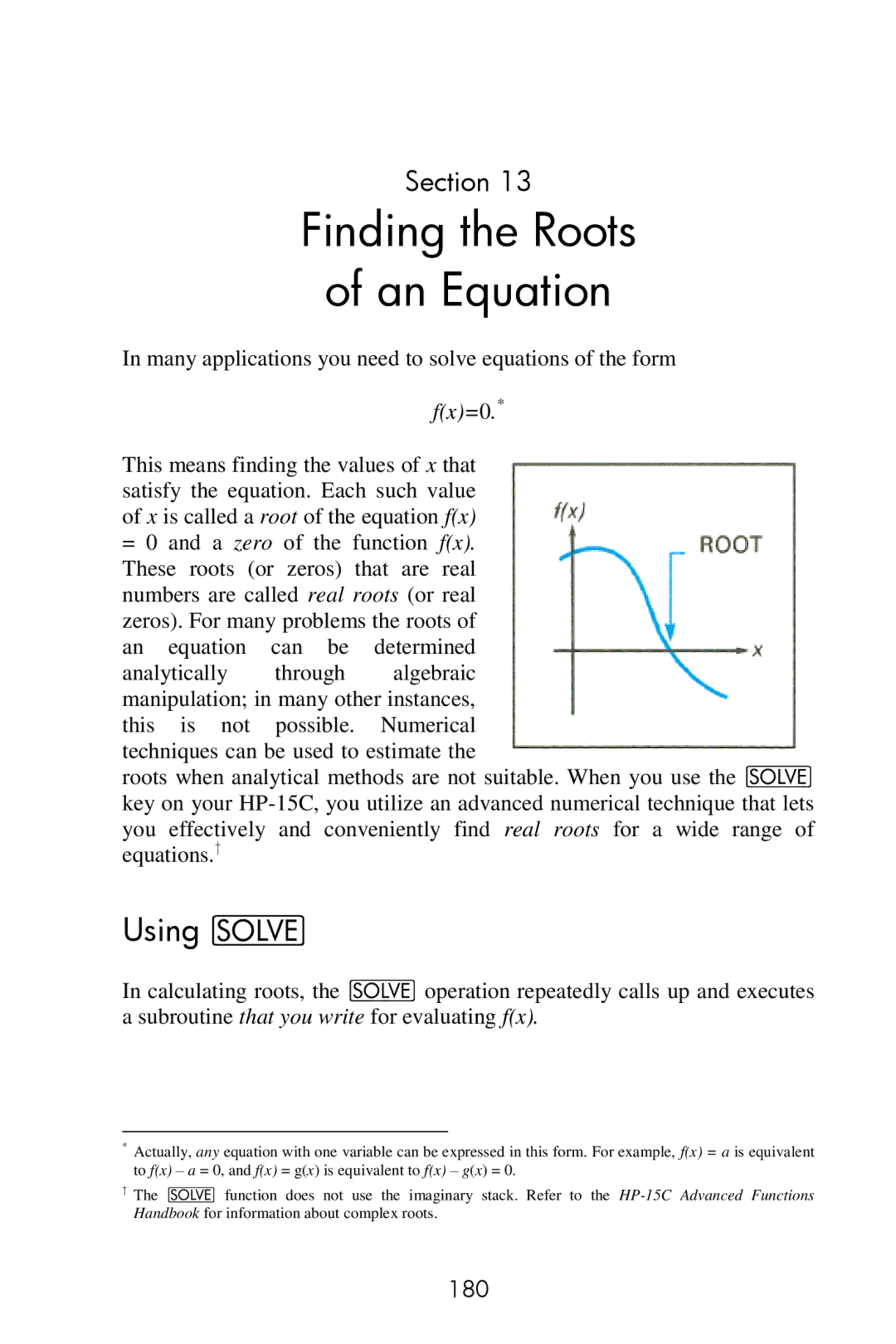
Finding the Roots An Equation
180
Finding the Roots of an Equation
Clear program memory
´b0
001-42,21
002 003
005 006 007
Finding the Roots of an Equation
Desired root
Keystrokes ¥
´ bA
000 001-42,21,11
003 004
5000 1 e t
Brings another t-value
Into X-register
200 t
When No Root Is Found
000 001-42,21 002 003 004 005
Error
Choosing Initial Estimates
Label
003 004 005 007
X + 8
008 009
6 x + 8
Finding the Roots of an Equation
Using in a Program
Restriction on the Use
Memory Requirements
Using f
Numerical Integration
194
002 003 004
4040
1416 7652
Begin subroutine with a label
3825
$ ÷
4401
6054
Accuracy of f
´ i ´ f
8826
7091
Using f in a Program
382
Memory Requirements
Error Conditions
Error 0 Improper Mathematics Operation
Appendix a
205
Error 1 Improper Matrix Operation
Error 2 Improper Statistics Operation
Error 3 Improper Register Number or Matrix Element
Error 4 Improper Line Number or Label Call
Error 5 Subroutine Level Too Deep
Error 6 Improper Flag Number
Pr Error Power Error
Stack Lift Last X Register
Digit Entry Termination
Stack Lift
Appendix B
Disabling Operations
Enabling Operations
Stack Stack Enabled. disabled 53.1301 No stack Lift
Neutral Operations
Appendix B Stack Lift and the Last X Register Keys
Nnn Clear u ¥
Last X Register
\ k + H ∆ \ h ÷ À P* q r c ‘ / N z ∕ P\ o j
Memory Allocation
Memory Space
Appendix C
Registers
Appendix C Memory Allocation
Memory Reallocation
Memory Status W
M % Function
Restrictions on Reallocation
´m% 1.0000 Whold 1 64
19 ´ m
Program Memory
Automatic Program Memory Reallocation
Memory Requirements for the Advanced Functions
Two-Byte Program Instructions
If executed
Together
Appendix C Memory Allocation
Detailed Look at
How Works
Appendix D
220
Appendix D a Detailed Look at
Accuracy of the Root
X4 =
000
1718
006 007 008 009 010-43,30 011 012-43,30 013
Interpreting Results
´ v B
0681
− 45 For 0 x
Test for x range
Branch for x ≥
3x 45x 2 +
End subroutine
000.0000
Initial estimates
1358
Possible root
Appendix D a Detailed Look at
´ b.0 001-42,21,.0 002 003 004 005
Bring x-value into X-register
007 008 009 010
013 014 015 016
017 018
10 v ´ ‛ 20
Error 0000 1250 5626
Finding Several Roots
Fx = xx a3 =
002 003 004 005 006 007
6667
Same initial estimates
Second root
Stores root for deflation
Deflated function value
Deflation for third root
Limiting the Estimation Time
For Advanced Information
Counting Iterations
Specifying a Tolerance
Detailed Look at f
How f Works
Appendix E
240
Accuracy, Uncertainty, and Calculation Time
X = π1 0π cos4θ − x sinθ dθ
0000 1416
´ i ´ f
Keystrokes Display Return approximation to
´ Clear u Hold
Keystrokes ´ i Display
´ f ´ Clear u hold
7858
7807
Uncertainty and the Display Format
Functions values for example
Δx = 0.5×10−n ×10m
= aδx dxb = ab 0.5×10−n + m x dx
Conditions That Could Cause Incorrect Results
∞ xe− xdx
001-42,21 002- 1 003 004 005
Appendix E a Detailed Look at f
Appendix E a Detailed Look at f
Conditions That Prolong Calculation Time
Keys lower limit into
Keys upper limit into
Approximation to integral
Uncertainty
Appendix E a Detailed Look at f
Obtaining the Current Approximation to an Integral
For Advanced Information
Low-Power Indication
Installing New Batteries
Batteries
Batteries
Appendix F Batteries
Verifying Proper Operation Self-Tests
2.C 3.H
Function Summary and Index
Complex Functions
Conversions
Digit Entry
Display Control
Index Register Control
Logarithmic Exponential Functions
Mantissa. Pressing
Mathematics
Matrix Functions
146
Number Alteration
To ZP page164
To XT
Percentage
Probability
Stack Manipulation
Clear u
Statistics
Storage
Trigonometry
Programming Summary and Index
269
Programming Summary and Index
Subject Index
271
Subject Index
Subject Index
Subject Index
Subject Index
Subject Index
Subject Index
Subject Index
Subject Index
Subject Index
Subject Index
Subject Index
Subject Index
Product Regulatory Environment Information
Federal Communications Commission Notice
Modifications
Canadian Notice
Avis Canadien
European Union Regulatory Notice
Body number is inserted between CE