By Galil Motion Control, Inc
DMC-2x00
Using This Manual
Contents
Connecting Hardware
Communication
Iv Contents DMC-2X00
Application Programming
Troubleshooting 130
Contents y
Index 210
Overview
Electrical Specifications
Specifications
Mechanical Specifications
DMC- 2000 Family Part Number Definition
Equipment Maintenance
Environmental Specifications
Standard Servo Motor with +/- 10 Volt Command Signal
Overview of Motor Types
Amplifiers in Current Mode
Stepper Motor with Step and Direction Signals
Amplifiers in Velocity Mode
Overview of Amplifiers
Microcomputer Section
DMC-2x00 Functional Elements
Motor Interface
Communication
System Elements
General I/O
Motor
Amplifier Driver
Encoder
Watch Dog Timer
This page Left Blank Intentionally
Getting Started
Outline of the DMC-2000 Daughter Board
DMC-2000 Daughter Board
3B Outline of the DMC-2200 Daughter Board
DMC-2200 Daughter Board
CABLE-USB-2M CABLE-USB-3M
Elements You Need
Recommended System Elements of DMC-2100/DMC-2200
Standard Servo Motor Operation
Installing the DMC-2x00
Determine Overall Motor Configuration
Master Reset and Upgrade Jumpers
Install Jumpers on the DMC-2x00
Sinusoidal Commutation
Stepper Motor Operation
Configure DIP switches on the DMC-2000
Switch 10 USB
Switch 4, 5 and 6 Main Serial Port Baud Rate
Configure DIP switches on the DMC-2100
Configure DIP switches on the DMC-2200
Connect AC Power to the Controller
Install the Communications Software
Communicating through the Main Serial Communications Port
Establish Communications with Galil Software
Communicating through the Ethernet
Communicating through the Universal Serial Bus USB
Determine the Axes to be Used for Sinusoidal Commutation
Sending Test Commands to the Terminal
Make Connections to Amplifier and Encoder
DMC-2X00
Connect Standard Servo Motors
BGA CR
Inverting the Loop Polarity
ICM-2900
Connect Sinusoidal Commutation Motors
Example Sinusoidal Commutation Configuration using a DMC
Getting Started DMC-2X00
SHA
Tune the Servo System
Connect Step Motors
System Set-up
Design Examples
InstructionInterpretation
Instruction Interpretation
Multiple Axes
Profiled Move
Independent Moves
Position Interrogation
Velocity Control
Absolute Position
Using the On-Board Editor
Operation in the Buffer Mode
Operation Under Torque Limit
Interrogation
Line # Instruction Interpretation
Motion Programs with Loops
Motion Programs with Trippoints
Linear Interpolation
Control Variables
XQ #B
AMA
LM ABC
Circular Interpolation
BGS
VM AB
This page Left Blank Intentionally
Overview
Using Optoisolated Inputs
Limit Switch Input
Abort Input
Home Switch Input
Reset Input
Wiring the Opto-Isolated Inputs
Uncommitted Digital Inputs
Opto-Isolation Common Point
Optoisolated Inputs
Using an Isolated Power Supply
Bypassing the Opto-Isolation
Analog Inputs
Amplifier Interface
Auxiliary Encoder Inputs
TTL Inputs
Output Compare
TTL Outputs
General Use Outputs
Interfacing to Grayhill or OPTO-22 G4PB24
Error Output
Extended I/O of the DMC-2x00 Controller
RS232 Ports
Introduction
RS232 Main Port P1 Dataterm
RS232 Auxiliary Port P2 Dataset
RS422 Main Port P1
RS-232 Configuration
RS422 Auxiliary Port P2
Baud Rate Selection
Address
Daisy-Chaining DMC-2000 only
Communication Protocols
Ethernet Configuration DMC-2100/2200 only
Command Interpretation
LED
Addressing
DMC-2X00
Communicating with Multiple Devices
Multicasting
Using Third Party Software
Data Record Map
Data Record
DMC-2X00
BIT
Header Information Byte 0, 1 of Header
Bytes 2, 3 of Header
Axis Switch Information 1 Byte
General Status Information 1 Byte
Axis Status Information 2 Byte
QZ Command
Controller Response to Commands
Galil Software Tools and Libraries
Unsolicited Messages Generated by Controller
Important All DMC-2x00 commands are sent in upper case
Command Syntax Ascii
BG Abcdefgh
BG Abcd
BG D
BG S
Header Format
Command Syntax Binary
Datafields Format
Binary Command Table
Example
LE, VE
Controller Response to Data
Summary of Interrogation Commands
Interrogation Commands
Interrogating Current Commanded Values
Interrogating the Controller
=TPA
Command Summary
This page Left Blank Intentionally
Example Application Mode of Motion Commands
VS,VA,VD
Independent Axis Positioning
GA, GD
GP, GR
Operand Summary Independent Axis
Command Summary Independent Axis
Multiple Move Sequence
Examples
Absolute Position Movement
20000
Position Tracking
15000 10000
5000 Time ms 100
Example Motion
Command Description PT1
Motion
Example
Position vs. Time msec Motion
Velocity vs.Time Motion
Velocity cts/sec vs. Time msec with IT Motion
Trip Points
Independent Jogging
Command Summary Position Tracking Mode
Command Summary Jogging
Joystick Jogging
Jog in X only
AS a
#JOY
Specifying Linear Segments
Linear Interpolation Mode
Specifying the Coordinate Plane
Specifying Vector Speed for Each Segment
Additional Commands
Changing Feed Rate
#ALT
Linear Interpolation Motion
Command Summary Linear Interpolation
Operand Summary Linear Interpolation
LM CD
Linear Move
Position D
Multiple Moves
Vector Mode Linear and Circular Interpolation Motion
Specifying Vector Segments
Command Summary Coordinated Motion Sequence
Compensating for Differences in Encoder Resolution
Trippoints
Tangent Motion
Operand
Operand Summary Coordinated Motion Sequence
Tangent Axis
BG ABC
Coordinated Motion
AM ABC
SB0
Ramped Gearing
Electronic Gearing
Velocity cts/sec vs. Time msec Ramped Gearing
Electronic Gearing Over a Specified Interval
Command Summary Electronic Gearing
MO Z
GA Z, Z
Electronic Gearing
Gantry Mode
Simple Master/Slave
GA,A
Electronic Cam
DMC-2X00
3000 2250 1500 2000 4000 6000 Master a
Command Description
Command Summary Electronic CAM
Electronic CAM
Operand Summary Electronic CAM
Specifying Contour Segments
Contour Mode
DT0CD0
336 288 240 192 Time ms
CMA
Command Description CM Abcdefgh
Command Summary Contour Mode
General Velocity Profiles
Generating an Array
#POINTS
Contour Mode
Record and Playback Example
Teach Record and Play-Back
Sinusoidal Motion Example
Virtual Axis
Ecam master example
Stepper Motor Operation
Monitoring Generated Pulses vs. Commanded Pulses
Specifying Stepper Motor Operation
Stepper Motor Smoothing
Motion Complete Trip point
Using an Encoder with Stepper Motors
Command Summary Stepper Motor Operation
Operand Summary Stepper Motor Operation
Stepper Position Maintenance Mode SPM
Example SPM Mode Setup
Error Limit
Correction
SHX
#MOTION
Example Error Correction
Example Friction Correction
Dual Loop Auxiliary Encoder
Backlash Compensation
Additional Commands for the Auxiliary Encoder
Continuous Dual Loop
= Main Encoder Second Encoder
Sampled Dual Loop
Motion Smoothing
#DUALOOP
DE0
Using the IT and VT Commands
Trapezoidal velocity and smooth velocity profiles
Using the KS Command Step Motor Smoothing
Homing
Home Sensor Home Switch
High Speed Position Capture The Latch Function
Command Summary Homing Operation
Operand Summary Homing Operation
Input Function
AL B
#LATCH
#WAIT
JP #WAIT,ALB=1
Application Programming
Using the DOS Editor to Enter Programs DMC-2000 only
ED #BEGIN
Edit Mode Commands
Program Format
Using Labels in Programs
Special Labels
#START
Commenting Programs
No Command
REM Half Circle Motion VP 0,3000 REM TOP Line
REM Command
Executing Programs Multitasking
Debugging Programs
Trace Commands DMC-2100/2200 only
RAM Memory Interrogation Commands
Error Code Command
Stop Code Command
Event Triggers & Trippoints
Program Flow Commands
AS a B C D E F G H
Command Function
DMC-2x00 Event Triggers
Example- Set Output after Distance
Example- Multiple Move Sequence
Example- Repetitive Position Trigger
Example Start Motion on Input
Example Change Speed along Vector Path
Example Set Output when At Speed
Conditional Jumps
Example- Define Output Waveform Using AT
Example Multiple Move with Wait
Logical operators
Command Format JP and JS
Conditional Statements
Multiple Conditional Statements
If, Else, and Endif
Examples
JP#A
#BEGIN
Using the Else Command
Using the if and Endif Commands
Command Format IF, Else and Endif
Nesting if Conditional Statements
Auto-Start Routine
Subroutines
Stack Manipulation
Automatic Subroutines for Monitoring Conditions
Example Limit Switch
Example Input Interrupt
Example Position Error
Example Motion Complete Timeout
Example Command Error
PAA=0
Example Command Error w/Multitasking
Example Communication Interrupt
Example Ethernet Communication Error
Mathematical Operators
Mathematical and Functional Expressions
Bit-Wise Operators
Operator Function
Response from command MG len6 S4
Functions
Variables
Assigning Variable Values to Controller Parameters
Programmable Variables
Assigning Values to Variables
Operands
Example Using Variables for Joystick
Displaying the value of variables at the terminal
Special Operands Keywords
Defining Arrays
Arrays
Assignment of Array Entries
Time
Automatic Data Capture into Arrays
Using a Variable to Address Array Elements
Uploading and Downloading Arrays to On Board Memory
Data Types for Recording
Command Summary Automatic Data Capture
Operand Summary Automatic Data Capture
Example Recording into an Array
Deallocating Array Space
Input of Data Numeric and String
Input of Data
Example- Inputting Numeric Data
Keyword Function
Operator Data Entry Mode
Using Communication Interrupt
Inputting String Variables
Output of Data Numeric and String
Formatting Messages
Sending Messages
Specifying the Port for Messages
Summary of Message Functions
Using the MG Command to Configure Terminals
Displaying Variables and Arrays
Example Printing a Variable and an Array element
LZ0
0000000009
Formatting Variables and Array Elements
Local Formatting of Response of Interrogation Commands
Local Formatting of Variables
LZ1
Converting to User Units
Hardware I/O
Digital Outputs
Alph
Example- Output Bit
Example- Set Bit and Clear Bit
Example- Output Port
Example Turn on output after move
Example Start Motion on Switch
Example Using Inputs to control program flow
Input Interrupt Function
AI 1BGA
Example Position Follower Point-to-Point
Analog Inputs
TP AB
ST AB
Example Position Follower Continuous Move
Configuring the I/O of the DMC-2x00
PA VP
#CONT
Saving the State of the Outputs in Non-Volatile Memory
Accessing Extended I/O
Bit I/O Block Binary Decimal Value for Representation
Argument Blocks Bits Description
Interfacing to Grayhill or OPTO-22 G4PB24
Wire Cutter
Example Applications
JP #A
Table Controller
BGS AMS
AMC
BGC AMC
Speed Control by Joystick
Backlash Compensation by Sampled Dual-Loop
Position Control by Joystick
BGA JP #B
This page Left Blank Intentionally
Output Protection Lines
Hardware Protection
Input Protection Lines
Software Protection
Automatic Error Routine
Off-On-Error
Programmable Position Limits
#AJP #AEN
Limit Switch Routine
Installation
Troubleshooting
Operation
Communication
Stability
Theory of Operation
Level
Velocity and Position Profiles
Operation of Closed-Loop Systems
Functional Elements of a Motion Control System
System Modeling
Current Drive
Motor-Amplifier
Voltage Drive
Elements of velocity loops
Velocity Loop
Voltage Source
Digital Filter
DAC
ZOH
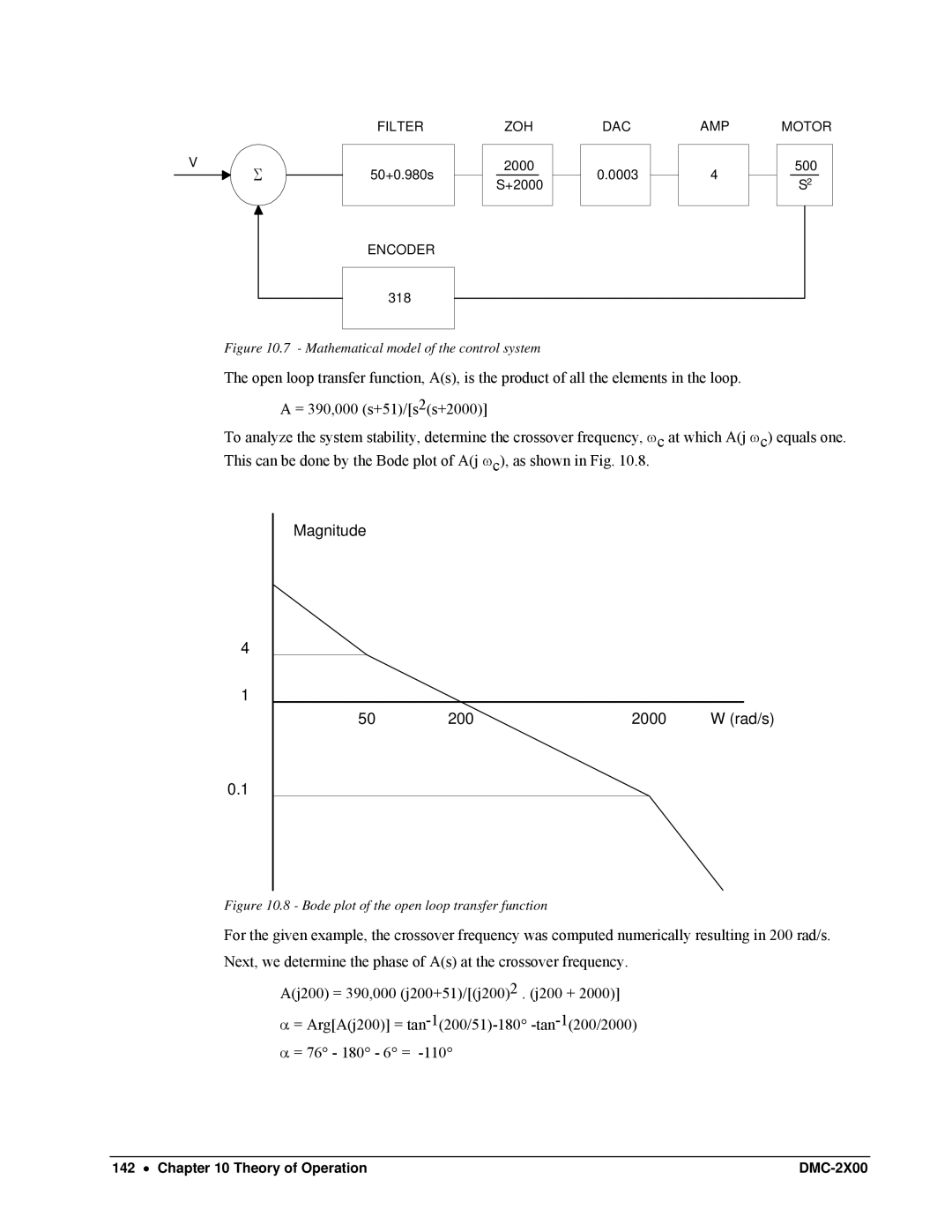
System Analysis
Magnitude 200 2000 Rad/s
Analytical Method
System Design and Compensation
Theory of Operation DMC-2X00
Equivalent Filter Form
Servo Control
Electrical Specifications
Stepper Control
Input / Output
Power
Performance Specifications
Minimum Servo Loop Update Time
Normal
Fast Update Rate Mode
DMC-2x00 Axes A-D High Density Connector
Connectors for DMC-2x00 Main Board
DMC-2x00 Axes E-H High Density Connector
DMC-2x00 Extended I/O 80 Pin High Density Connector
DMC-2x00 Auxiliary Encoder 36 Pin High Density Connector
+5V
RS-232-Main Port
Pin Signal
RS-232-Auxiliary Port
USB USB Out
Pin Serial Connector Male, D-type
Standard RS-232 Specifications
Cable Connections for DMC-2x00
Ethernet
Pin Male computer Pin female controller
DMC-2x00 Serial Cable Specifications
Pin Female terminal Pin Male Controller
Pin Female Computer Pin Female Controller
Pin Male terminal Pin male controller
Inputs
Pin-Out Description for DMC-2x00
Outputs
Appendices DMC-2X00
Function If jumpered
Jumper Description for DMC-2x00
Jumper Label
Dimensions for DMC-2x00
DMCWIN16 DMCWIN32 SETUP16 SETUP32 KIT
Accessories and Options
Block 4 PIN Label Description
ICM-2900 Interconnect Module
Description
Pwmx
Error
Mocmdy
Signy
IN8
Reset
Xlatch
Ylatch
MAZ
+MAZ
+MBZ
MBZ
ICM
ICM-2900 Drawing
ICM-2908 Interconnect Module
ICM-2908
ICM-2908 Drawing
PCB Layout of the ICM-2900
ISO OUT Power Error Reset CMP Mocmdw
Features
ICM-1900 Interconnect Module
Terminal Label
DMC-2X00
100 101 102 103 104 105 106 107
MBW +INW
AMP-19x0 Mating Power Amplifiers
ICM-1900 Drawing
Standard Opto-Isolation and High Current Opto-isolation
Specifications
Opto-Isolated Outputs for ICM-2900 / ICM-1900 / AMP
Changing the Amplifier Enable Voltage Level
Configuring the Amplifier Enable for ICM-2900 / ICM
Laen Option
DMC-2000 ICM-1900 / ICM-2900
Overview
IOM-1964 Opto-Isolation Module for Extended I/O
Figure A-7
Configuring Hardware Banks
Input Circuit
Figure A-10
High Power Digital Outputs
Figure A-12
Standard Digital Outputs
Figure A-14
Digital Inputs
Relevant DMC Commands
High Power Digital Outputs
Standard Digital Outputs
Screw Terminal Listing
DMC-2X00
PWROUT31
PWROUT32
PWROUT30
PWROUT29
CB-50-100 Adapter Board
Connectors
Appendices DMC-2X00
JC6 50 PIN IDC J9 100 PIN High Density Connector
JC8 JC6
CB-50-100 Drawing
Figure A-16
CB-50-80 Adapter Board
JC8
JC6
CB-50-80 Outline
CB-50-80 Drawing
CB-50-80 Layout
Figure A-19
TERM-1500 Operator Terminal
Figure A-20
Specifications Hand-Held
Keypad Maps Hand-Held
Specifications Panel Mount
Single Key Output
Shift Key Output
Keypad Map Panel Mount 6 columns x 5 rows
Escape Commands
Cursor Movement Commands
Configuration
Default Function Keys
Default Configuration
Input/Output of Data DMC-2x00 Commands
Function Keys
Ordering Information
Pin Modular Connector
Pin D Adaptor Male For P2
Vs = Vx 2 + Vy
Coordinated Motion Mathematical Analysis
Lk = Xk 2 + Yk
Velocity
Example- Communicating with OPTO-22 SNAP-B3000
MODULE=2
#CFGDOUT
CFGVALUE=$180
NUMOFIO=4
Appendices DMC-2X00
DMC-2x00 DMC-1500
DMC-2x00/DMC-1500 Comparison
Training Seminars
List of Other Publications
Contacting Us
Warranty
Limswi
Index
PID
Poserr