LBA-PC
OPERATOR’S Manual
LBA-PC
Optical Radiation Hazards Electrical Hazards
Equipment Setup
Installation of the Frame Grabber Board Camera Connections
For Windows 2000 and Windows XP Pro
Menus and Dialog Boxes
Capture... define acquisition method and processing
Camera... selection and display resolution
Capture Toolbar... design the toolbar contents
Aperture... display and define apertures
Display Windows
Triggering Types & Capturing Methods
Digital Camera Control
What is Ultracal
Computations
Integration Operation
Remote Operation
Digital Camera Option
Active X
Remote Gpib Operation
System Requirements
General Information
Optional Equipment
Power Requirements
Specifications
Optical Radiation Hazards
Safety Considerations
Electrical Hazards
LBA-7XXPC -D LBA-PC-PIII
Equipment Setup
Installation of the Frame Grabber Board
LBA-300PC
LBA-PC
Four Camera Option Operator’s Manual
Camera Connections
First Method Windows XP
LBA-PC Software Installation
Analog Cameras
Digital Cameras
Second Method Windows 2000 or XP
Start LBA-PC
Start, Programs, Spiricon, LBA-PC, LBA-PC
Sample Configurations
Configure Camera Type
Collect Data
LBA-PC device driver not found LBA-PC set to Off-Line mode
Error Messages
Unable to load LCA program file File Not Found
Click on the Sound, video and game controllers listing
Connections
Frame Grabber not found LBA-PC set to Off-Line mode
Not a valid LBA-PC data file
Shutter Controls Signals
Camera Power
SHUT3 SHUT2 SHUT1
Trigger
Camera Control Cables
Trigger Out
Pass/Fail Out
LBA-300/400/500/708/710/712/714PC Digital camera option
Special Setup for Pyrocam I Operation
Pyrocam I Setup Requirements
Pyrocam I with Non-Digital LBA-PC’s
Some Restrictions apply when interfaced to a Pyrocam
Setup requirements for LBA-PC with pyrocam cameras
Image synchronization considerations
Pyrocam I with Digital LBA-PC’s
LBA-500/7XXPC-D w/ Digital Camera Option
LBA-500/7XXPC-D Setup requirements
Image Synchronization Considerations
File Load
File. . . Drop Down Menu Selections
Load Frame Dialog Box
Special Frame Numbers
File Save As…
Drag and Drop Data Frame Loading
Save As … Dialog Box
Export Image… to a disk file
Export Image… dialog box
Restore Config… from a file
Save Config… to a file
Set Reference, copy the current to the reference frame
Generate Gain
How to generate gain
What is Gain Correction?
What Disables Gain Correction?
File Logging
File Load Gain…
File Save Gain As…
Gain Off/On
Data, Results & Export Logging, dialog box
Beginning and Terminating Logging
Logging Method
Pass/Fail Filter
Combinations using Logging, Statistics and Post Processing
Combinations using Logging, Statistics and Block Mode
File Print…
File Exit
File Print Setup
File Save Frog as…
Save Frog as…Dialog Box
Frog Data Collection Tips
Frog Data Orientation
LBA-PC
Aperture Shapes
Options... Drop Down Menu Selections
Hide/Show Capture, Display, Aperture Toolbar
Aperture... display and define apertures
Drag and Drop Apertures
How to create a Drawn Aperture
Display Beam Width
Using Auto Apertures
Camera type selection
Camera... selection and display resolution
Creating a new Camera Type
Click on Save CAM
Resolution and Frame Size
Frame Buffer Size
Sync Source
Am I using Virtual Memory yet?
Gamma Correction
Pixel Scale, Pixel Units
Capture... define acquisition method and processing
Special Camera Settings
Lens
Capture
LBA-PC
Camera
Capture Interval
LBA-PC
Trigger
LBA-PC
Processing
Reference Subtraction
Frame Summing
Frame Average
Gain Correction
Capture Toolbar... design the toolbar contents
Write Protect
Computation... Energy calibration select results items
Logging
Print
Min
Energy Calibration Procedure
Quantitative display, on/off
Total energy Aperture
Beam Width Method
LBA-PC
Gauss Fit
Elliptical
Top Hat
Divergence
Histogram
Statistics
Beam Display... define the beam display
Cursors
Beam View
Cursor Orientation
Origin Location
Axis Scale
Beam Colors
Beam Display
Set Reference Source
Color Bar
Display Thresholds
Copy Image to Wallpaper
Copy Image to Clipboard
7.13 2D Only Beam Display Items
7.14 3D only
LBA-PC
Beam Stability
Beam Display Toolbar
Main Controls
Strip Chart Controls
LBA-PC
Peak/Centroid Scatter Plot and Histogram
Options Camera
Options Camera Pixel Units
Options Beam Display
∝ m
LBA-PC
Option Camera
Create Palette…
LBA-PC
Save Colors
Saving the Palette
Clearing Colors
Load Colors
Pass/Fail... enable and define its operation
Password Lockout
Pass/Fail... Drop Down Menu Selections
Pass/Fail dialog boxes
Quantitative Pass/Fail
Pass or Fail results
Pass/Fail Units
Centroid
Elliptical Pass/Fail
Gauss Pass/Fail
Top Hat Pass/Fail
Top Hat Fluence
Window... Drop Down Menu Selections
Tile
How to Ultracal
Start!/Stop!... a Toggle Menu Action Item
Ultracal! Menu Action Item
AutoExposure Interacts with Ultracal
What Disables Ultracal
AutoExposure!… Menu Action Item
AutoExposure! Operation
Operator’s Manual 104
Operator’s Manual 105
Beam Display Window
Main Window
Frame Comment
LBA-PC
Shortcuts
Results Display Window
Shortcuts
Hardware Zooming
Pan/Zoom Display Window
Analog Camera Zooming
Panning
Soft Zooming
Digital Camera Zooming
Zooming and Panning Constraints
Tilt/Rotate Display Window
Histogram Display Window
Shortcuts using the Mouse
Operator’s Manual 117
Triggering the LBA-PC
Triggering Types & Capturing Methods
Non-interlaced Cameras
Trigger Type CW
Type Trigger Out
Interlaced Cameras
Cmos Camera, Interlaced
Trigger Delay
CCD Frame Transfer Camera, Interlaced
CCD Interline and Full Frame Transfer Camera, Interlaced
Type Video Trigger
Type Trigger
With Trigger Type set to CW
Capture Methods and Rate Control
Programming the Capture Interval
CCD Frame and Interline Transfer Cameras, Interlaced
With Trigger Type set to Trigger Out
Integration Control
Digital Camera Binning Effects
Digital Camera Operations
Integration Operation
Digital Camera Control
Digital Camera Exposure Controls
Digital Camera ROI Formating
Digital Camera Triggering
Digital Camera Gain and Black Level Control
Operator’s Manual 127
Numerical Formats
Computational Accuracy
Beam Presentation Affects Results
What is Ultracal
Manual Background Energy Nulling
Clip Level
What is the Clip Level and how is it used?
Total Energy
Centroid Location
Percent in Aperture
Peak and Min
Peak Location
11.1 D4-Sigma Method
Beam Widths and Diameters
Knife Edge Method
= ∑x ∑y y − y2 ⋅ Zx, y
Percent of Energy Method
Elliptical beam
Percent of Peak Method
Whole Beam fits Line fits are X/Y or Major/Minor aligned
Gauss Fit
15 X/Y or Major/Minor line fit equations
Whole Beam fit equations
Correlation of Fit
Deviation of Fit
Top Hat
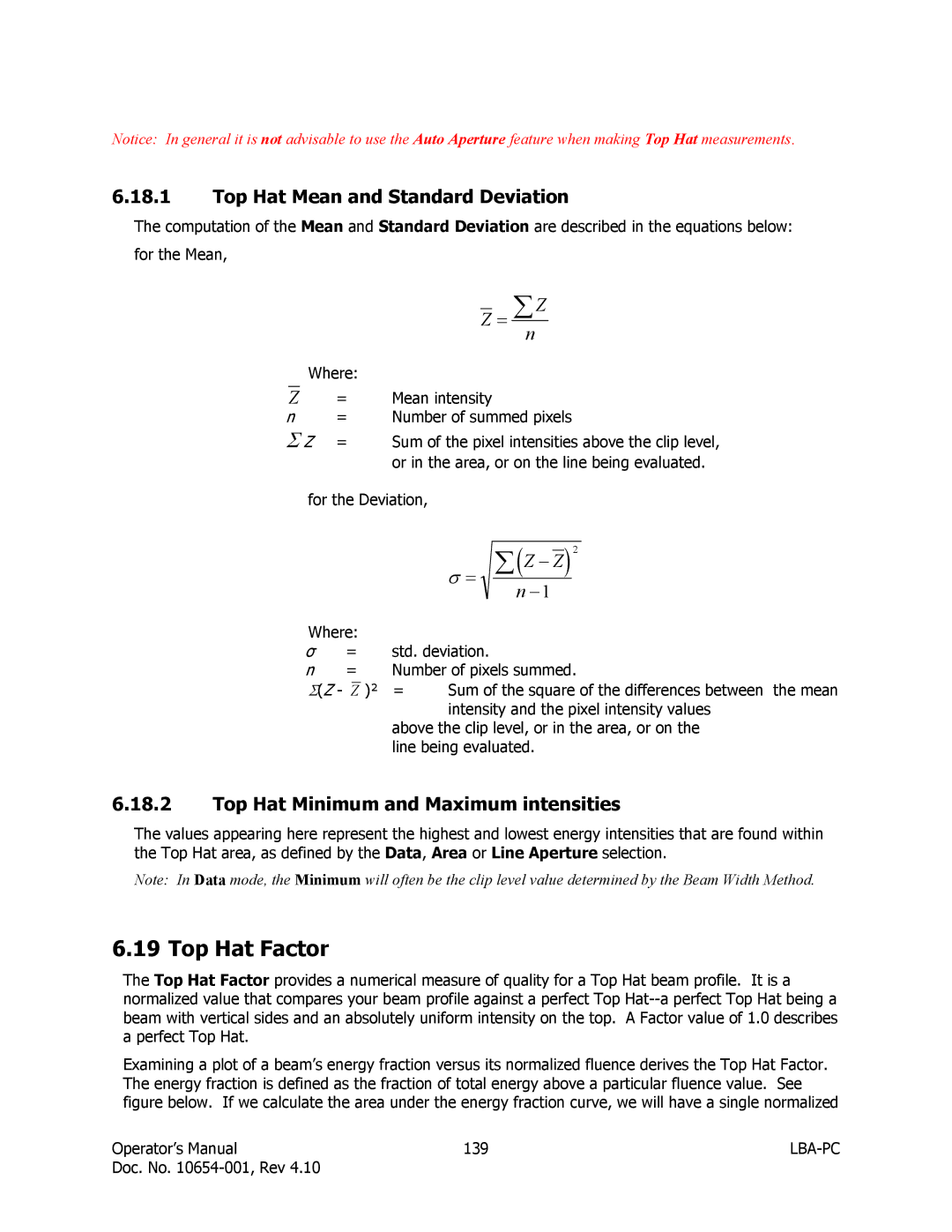
∑ Z
Top Hat Mean and Standard Deviation
Top Hat Factor
Top Hat Minimum and Maximum intensities
= ∑f i ⋅ NPix = Pk Total
Far-Field Divergence Angle computations
Effective Area and Effective Diameter
Focal Length Method
Far-Field Method
Histogram
Statistics
∑ S
Frame Summing
Frame Averaging
Z 1/ g
Gamma Correction
Convolution
Operator’s Manual 147
I/O Connections
Digital Camera Option
Digital Camera Connections for LBA-7XX Model Frame Grabbers
VD11-0+/-… LBA-400/500
Vsync
Special Jumper Cutting Requirements LBA-400/500 only
VD15-0+/-… LBA-7XX
SHT#… LBA-400/500 only
RST… LBA-400/500 only
Vertical Start
Digital Camera Advanced Timing Setup
Transfer Mode
Scan Mode
Horizontal Start
Vertical Size
Horizontal Size
Digital Camera and Ultracal Operation
LBA-PC
Remote Operation
How to Disable Remote Operation
Operator’s Manual 157
Introduction
Using ActiveX
Microsoft Excel
Visual Basic Visual Studio
LabVIEW
Properties, Methods, and Events
OperationComplete
Properties
AppInfo
Running
PixelHScale, PixelVScale
OperationError
NewFrame, HoldNewFrame
FrameData, FrameWidth, FrameHeight
Bitmap
CrosshairX, CrosshairY, CrosshairZ
CursorDelta
EnergyOfBeam
Quantitative Results
Results
Gauss Fit Results
Elliptical Results
Top Hat Results
Statistics Results
Divergence Results
Pass/Fail Results
Methods
LoadConfig
Open
Ultracal
OpenIndex
Start
Stop
Auto Exposure
Events
OnNewFrame
True
Dcom
OnOperationComplete
False
Server LBA-PC Computer
Remote Access
Client Application Computer
If you have a problem
LBA-PC
Hardware and Software Requirements
Remote Gpib Setup
AT-GPIB/TNT PCI-GPIB PCI-GPIB+ PCMCIA-GPIB PCMCIA-GPIB+
LBA-PC
Ieee 488.1 Command Support
Command Formats and Responses
LBA-PC Command and Data Formats
Ieee 488.2 Common Commands
DAB
Establishing Remote Control
SDC Save Config…
Configuration Commands
Restore and Save Configuration Files
LDC Restore Config…
Configuration Commands
APT?END
Minor=7.500E+00 Rotation=0 DisplayShape=0 AutoAperture=1
Transferring Raw Data
Transfer Commands
DAW
10.6.1.1 RCC?, RCR? Read Cursor Transfer
RCR?END
10.6.1.2 RDD? Read Frame Transfer
RDD?END
Transferring Data Files
LDD
10.6.2.1 FRM? Download Data Frame
FRM?
FRM
FRM
FRM Upload Data Frame
FrameNumber=33 #6124928DAB…DABEND
LDD Read Data File
SDD Save Data File
10.6.2.5 RDR? Read Results
RDR?
LOG Logging
10.6.2.7 FST? Transferring Status Information
FST?
10.6.3 PFS? Pass/Fail Status
Spatial Coordinates
Coordinate Systems
10.7.2 Pan/Zoom Window Detector Coordinates
PAN Set Capture Window Location
DIS Set Manual Origin Location
PAN?END
Frame Coordinates
Beam Window World Coordinates
Error Messages
LDC
LDC cannot load config while runningEND
ELR
Service Request Response
STB
ESR
Operator’s Manual 210
UNL
Ieee 488.1 Command Support
Command Meaning Usage
Ieee 488.2 Common Commands
Return ESE contents
Bit 5 Event Status bit, ESB, set
ESE, *ESE? ESR?
Service Request Generation
Type Description
LBA-PC Command and Data Formats
Configuration Command Parameters
CCC =
Key Type Value Description
LBA-PC Configuration Commands
LDC restore configuration
File Menu
LDD load data from file
SDC save configuration
SDD save data to file
Key Type Value Description
LOG set logging configuration
EXP set export configuration & export images
BMP
FRM and RDD are ignored if the LBA-PC is Not in remote
GAI3
PRN set print configuration and print
GAI generate gain frame
REF set reference
Options Menu
APT set aperture configuration
CAM set camera configuration
CAP set capture configuration
Key Type Value Description
Key Type Value Description
PAN
COM set computations configuration
Operator’s Manual 230
DIS set display configuration
Key Type Value Description
EnergyOfBeam=0 then the range is 0 to
Pass/Fail Menu
PSW enter password
PFF set pass/fail master configuration
Pass/fail quant configuration
Key Type Value Description
Pass/fail gauss configuration
Pass/fail elliptical configuration
Key Type Value Description
Key Type Value Description
Pass/fail top hat configuration
Key Type Value Description
3.6 Pass/fail divergence configuration
Remote Specific Commands
CAL ultra cal
CUR?
CHR read/write cross hair location
CUR read/write cursor location
CHR?
DSF display frame
ERR error reporting
ERR?
Rangeerror
Message Error Type Description
Executionerror
Commanderror
Executionerror SDD
Executionerror LDD, SDD, DIS
Executionerror LDD, DIS
Executionerror LDD, SDD
REM
Executionerror CHR
Message Generated By
Message Generated By
Message Generated By
Message Generated By
FRM upload/download a data frame
FST frame status information
HHMMSS.DD
MM/DD/YY
LOC go to local
4.10 PAL? read color palette
ORG set manual origin to cursor location
PAN pan left/right/up/down
PAN?
PNW pan window limits
PNW?
4.14 RCC? read cursor column
4.13 PFS? read pass/fail status
PFS?
4.15 RCR? read cursor row
Key Type Value Description
LBA-714PC
4.16 RDD? read raw data
RDR
4.17 RDR? read results
STT start/stop toggle
REM go to remote
RUN start running
STP stop running
ZOM zoom in/out
4.23 WLD? read current frame boundaries
WLD?
ZMM?
Footnotes
ZMM zoom information
ZOM?
Operator’s Manual 264
\ Spiricon \ Lbapc
LBA-PC Remote Control Capabilities
Virtual Instrument VI Examples
VI Libraries
Display Beam Frame
Basic SubVI Library Examples
Auto Aperture ON/OFF
Comma String to Array
Get Frame Status Info
Do Ultracal
Frame SRE
Get Basic Results
Get Version
Get Palette
Get Pan Location
Get Tophat Results
Read Cursor Column/Row
Move Cursor
Move Pan
Read Basic Cursor info
Run Stop
Restore Configuration
Read Divergence Results
Results SRE
Set Energy and Units
Save Configuration
General VI Examples for the LBA-PC
Semicolon String to Array
Basic Results Panel
Basic Results
Beam Viewer Panel
Beam Viewer
Load Data
Basic Divergence
Basic Logging
Basic Tophat
Hotkeys
Get Data
Operator’s Manual 276
Index
Percent of energy
Convolution 66, 128
LabVIEW 160
Shortcuts 109, 110