HP 12C manual Power Curve Fit

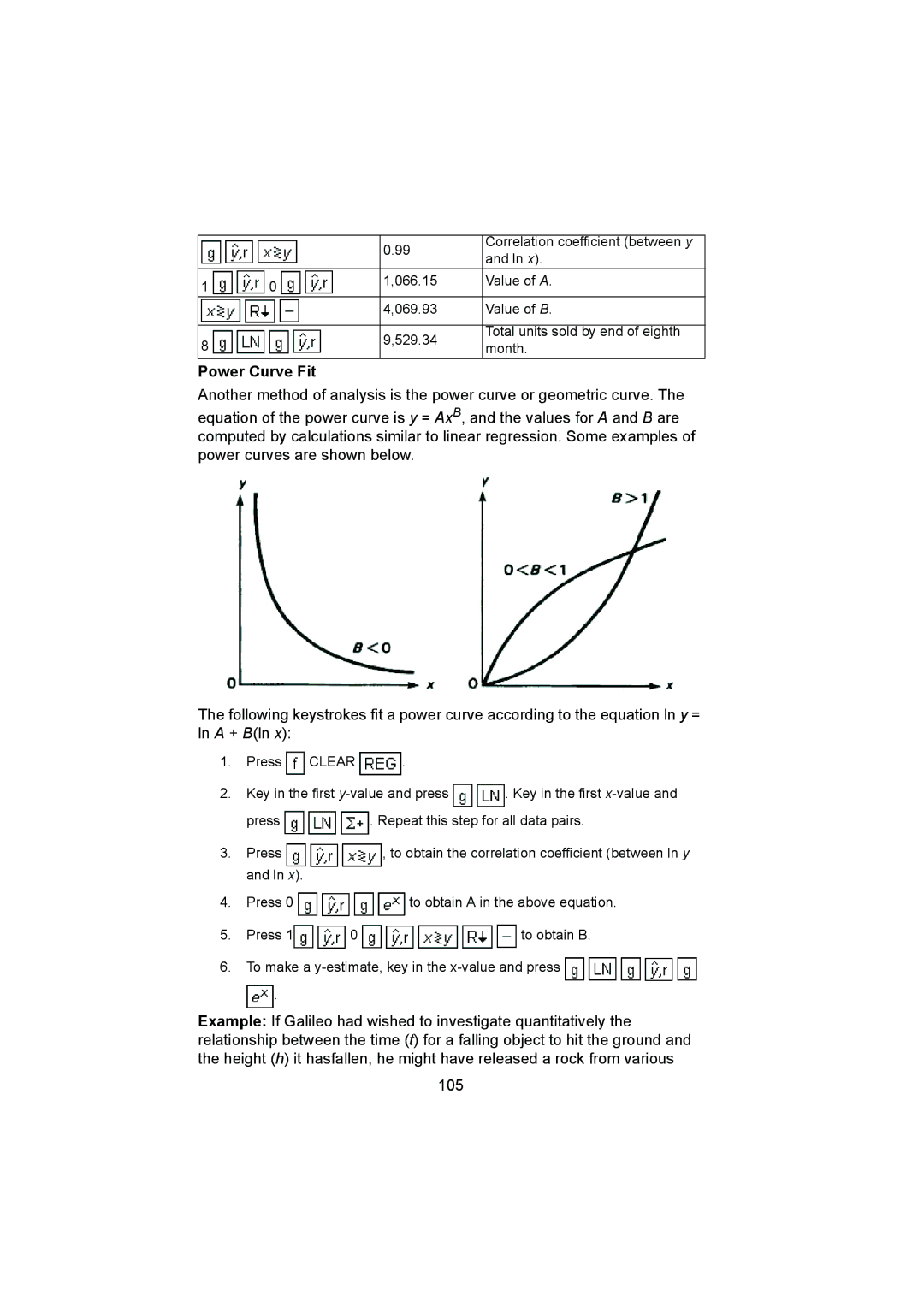
0.99Correlation coefficient (between y and ln x).

1	0	1,066.15	Value of A.
		4,069.93	Value of B.
8		9,529.34	Total units sold by end of eighth
			month.

Power Curve Fit

Another method of analysis is the power curve or geometric curve. The

equation of the power curve is y = AxB, and the values for A and B are computed by calculations similar to linear regression. Some examples of power curves are shown below.

The following keystrokes fit a power curve according to the equation ln y = ln A + B(ln x):

1.Press CLEAR .

2.Key in the first y-value and press . Key in the first x-value and

press . Repeat this step for all data pairs.

3.Press , to obtain the correlation coefficient (between ln y and ln x).

4.Press 0 to obtain A in the above equation.

5.Press 1 0 to obtain B.

6.To make a y-estimate, key in the x-value and press

.

Example: If Galileo had wished to investigate quantitatively the relationship between the time (t) for a falling object to hit the ground and the height (h) it hasfallen, he might have released a rock from various

105