8-13
Two-way Linkwitz-Riley Crossovers
Linkwitz-Riley designs constitute a special, even-order in-phase class of two-way sum-to-allpass response crossovers, and they must be configured in accordance with very strict phase and polarity requirements.
A properly implemented 2-way, 12 dB/octave Linkwitz-Riley crossover is characterized by:
•2nd-order Linkwitz-Riley (Butterworth-Squared) lowpass filter, -6 dB magnitude response at crossover frequency
•2nd-order Linkwitz-Riley (Butterworth-Squared) highpass filter, -6 dB magnitude response at crossover frequency
•polarity of highpass band opposite that of lowpass band
A properly implemented 2-way, 24 dB/octave Linkwitz-Riley crossover is characterized by:
•4th-order Linkwitz-Riley (Butterworth-Squared) lowpass filter, -6 dB magnitude response at crossover frequency
•4th-order Linkwitz-Riley (Butterworth-Squared) highpass filter, - 6 dB magnitude response at crossover frequency
•polarity of highpass band the same as that of lowpass band
Summarizing: In a 2-way, 12 dB/octave Linkwitz-Riley crossover the high band must be out-of-phase with the low band; in a 2- way, 24 dB/octave Linkwitz-Riley crossover the high band must be in-phase with the low band.
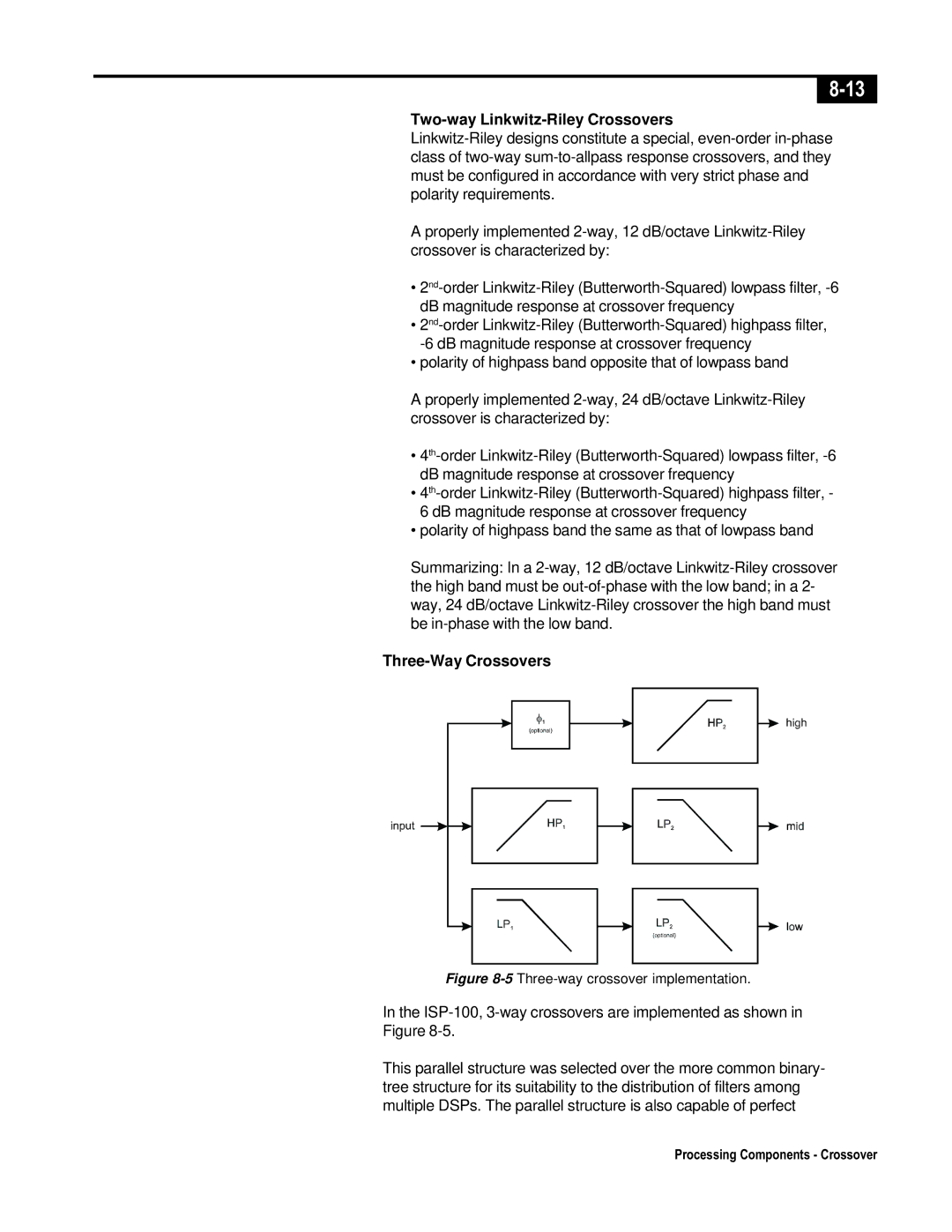
Three-Way Crossovers
Figure 8-5Three-way crossover implementation.
In the ISP-100, 3-way crossovers are implemented as shown in Figure 8-5.
This parallel structure was selected over the more common binary- tree structure for its suitability to the distribution of filters among multiple DSPs. The parallel structure is also capable of perfect